How to Select the Best ADC for Radar Phased Array Applications - Part 2
How to Select the Best ADC for Radar Phased Array Applications - Part 2
2023年06月15日
Read other articles in this series.
Abstract
Many papers discuss the system trade-offs and relative merits of digital vs. RF beamforming, and the hybrid blend thereof.1 Building on prior work, this article uses RF-to-ADC cascade modeling to show dynamic range (linearity and noise) and sample rate trade-offs against DC power consumption in a multichannel system with varying channel summation in both the RF and digital realms. The optimal selection of sample rate, ADC ENOB, and RF vs. digital channel combining is weighed against DC power consumption. The popular Schreier and Walden ADC figures of merit (FOMs) are proposed as extensible to a multichannel system to express a single system FOM portraying optimal dynamic range normalized for DC power expense. The article has two parts. “How to Select the Best ADC for Radar Phased Array Applications—Part 1” explains the method of modeling the system, and Part 2 analyzes the results and draws conclusions from system FOM.
System Modeling Results
The system model results presented in the following plots consist of:
| Merit Attribute | Swept Variables |
| SFDR | ADC ENOB; Blend of RF sum to digital sum, always totaling 64 |
| Sensitivity | |
| DC Power/Channel |
The following examples use a subarray size of 64 channels. In many of the plots, the horizontal axis shows the model sweep from all-digital summation on the left (64-channel digital sum, no RF sums) to all-RF summation on the right (no digital sum, 64-channel RF sum). In between is a digital and analog summation blend, sometimes called hybrid beamforming with increasing RF sum from left to right. These plots are in the next section. ADC ENOB is swept in the analysis and presented in the plots. Trends in DC power and performance (SFDR and SENS) are analyzed as these parameters are swept.
Figure 1 and Figure 3 are the parametric results from the system model. The trouble is, viewing sensitivity, SFDR, and DC power individually doesn’t indicate good or bad because performance is portrayed separately from power. For example, maximum SFDR at the lowest possible DC power burn is the goal. But what configuration of ADC ENOB and RF: digital combining is better vs. another? The next section is a more useful apples-to-apples comparison as the results show performance as dynamic range normalized for DC power burn.






Figures 4, 5, and 6 show the relative percentage of power consumed by the RFFE, ADC, and digital summation/interface. At every-element digital (Figure 4) and lower bit resolution, the digital interface and summation consume a large proportion of overall power. But for systems with higher RF channel summations, the digital interface is less significant. Another trend is that the RFFE is dominant at low ADC bit-resolution, and the ADC is dominant at high ADC bit-resolution. These plots show the big impact ADC ENOB and RF: digital channel summation ratio has on what dominates DC power consumption.






Next, the relative merit of SFDR and sensitivity is normalized for DC power/channel for different ADC ENOB and RF: digital channel sum schemes. The three independent plots of sensitivity, SFDR, and DC power vs. RF: digital channel summation from the prior sections is rearranged in the following figures to better visualize performance trends when normalized for DC power. Figure 7 and Figure 8 show SFDR and sensitivity vs. DC power for a few fixed RF: digital summation traces. The bit resolution is annotated and varies along the trace. Figure 9 and Figure 10 show the same underlying data but arranged a bit differently. The traces are a few ENOB cases, with the RF: digital summation varying along the trace from all-RF to all digital, left to right. Table 3 summarizes the conclusions from the plots.
| Attribute | Swept Variable | Markers on Each Trace |
| SFDR; sensitivity | DC power/channel Blend of RF to digital sum, always total = 64 |
ENOB increasing left to right 4b to 12b by 2b |
| SFDR; sensitivity | DC power/channel ADC ENOB | Blend of RF: digital sum, total = 64. 64:1 all-RF sum on left, going 32:2, 16:4, 8:8, 4:16, 2:32, ending 1:64 all-digital on right |








We can now draw performance vs. DC power conclusions from the plots:
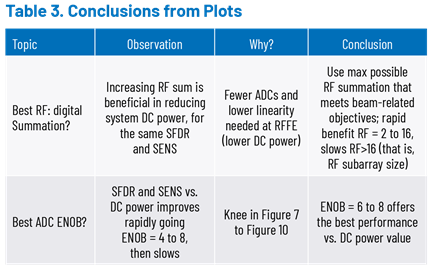
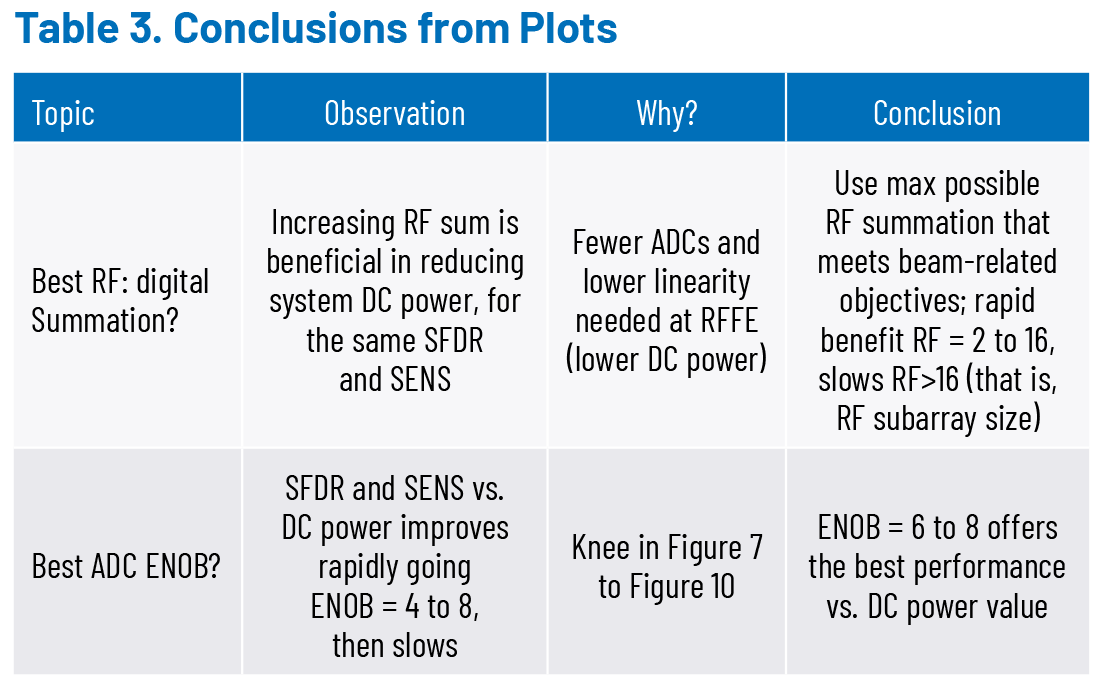
In general, for the same SFDR and sensitivity, hybrid beamforming systems employing a blend of RF and digital beamforming require a higher bit ADC vs. all-digital but are more power efficient overall. The knees in the plots show that there exists a sweet spot of performance when SFDR, sensitivity, and DC power burn are evaluated together. Diminishing returns exist as ENOB improves beyond the knee, and things deteriorate fast as ENOB degrades from the knee. Is every-element digital better in any of these situations? From a dynamic range efficiency perspective where DC power is handicapped, no. The benefits of every-element digital are in software-defined beamforming flexibility and adaptability. Extra power is burned to achieve this.
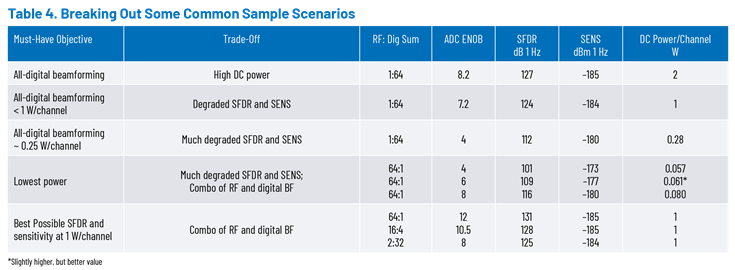
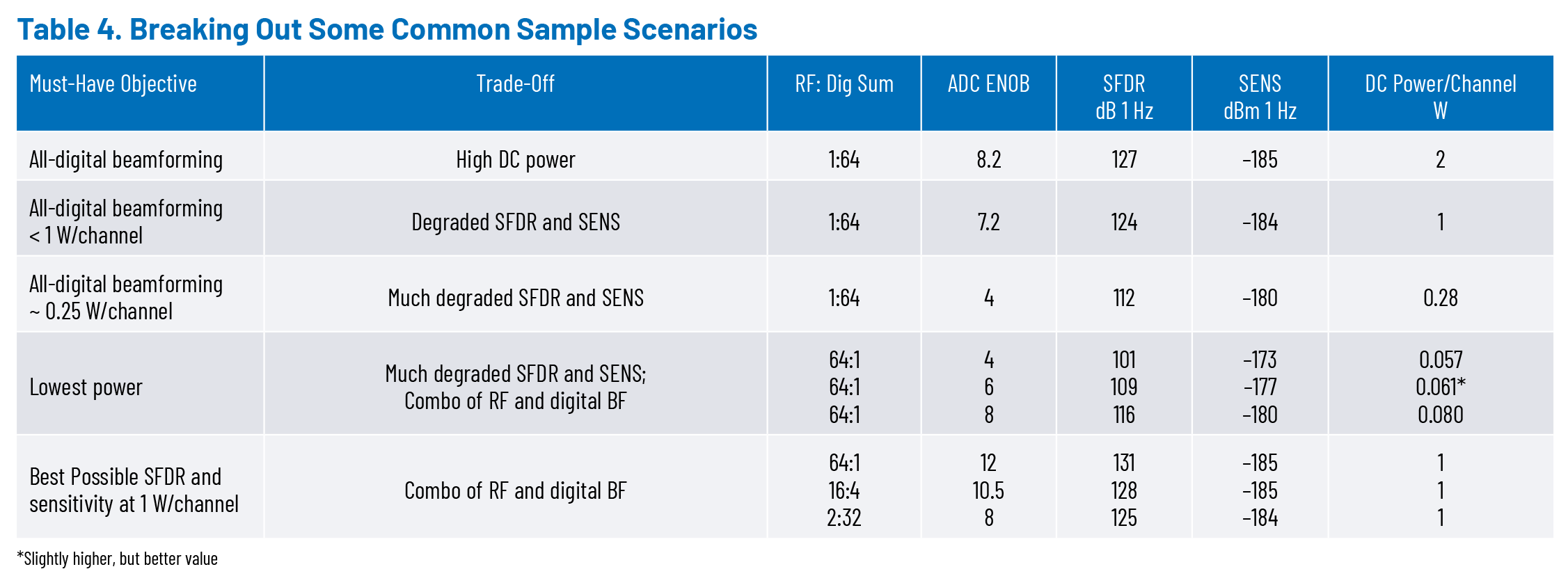
Table 4 provides a comparison of example scenarios each with a different design priority. There is no one-config-fits-all scenario. Different system objectives drive different performance priorities, which force performance trades across other attributes.
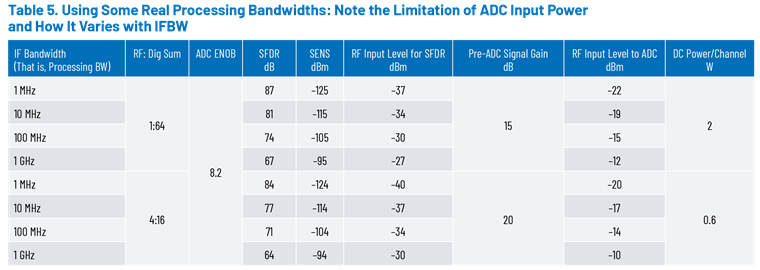
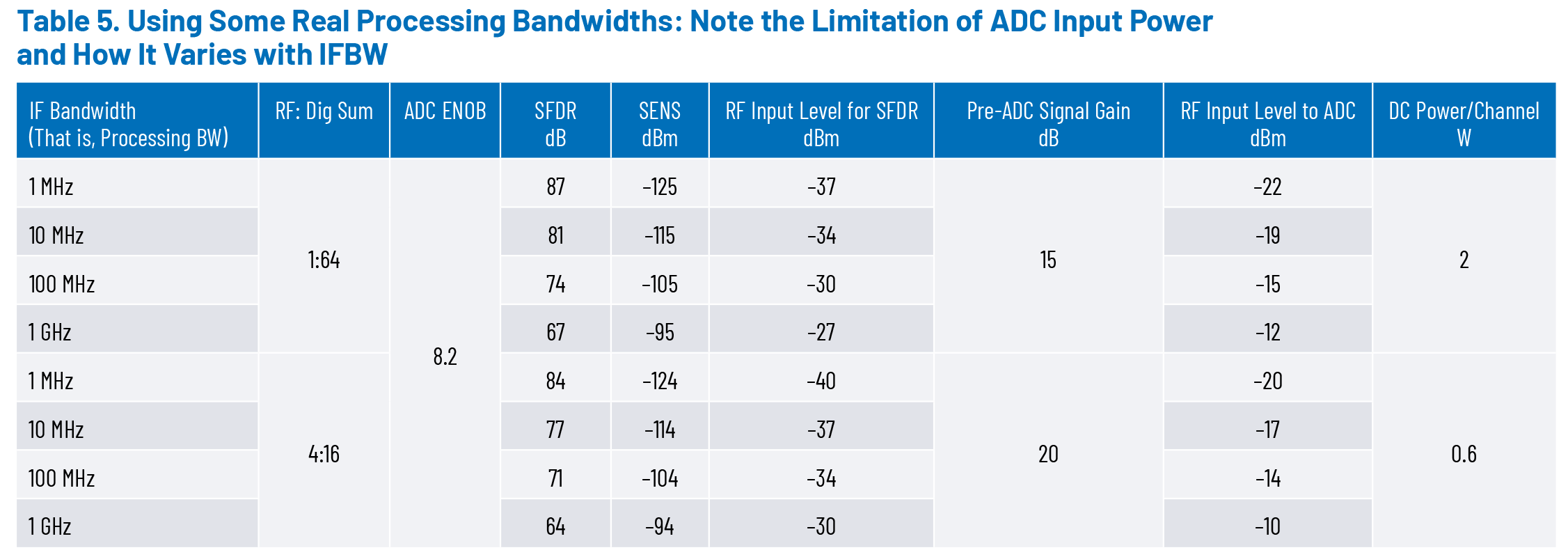
Table 5 shows a popular ADC ENOB = 8 example assuming practical IF bandwidths. Pay attention to the signal level at the ADC, as the linearity of most ADCs degrade as full scale is approached. The optimal RF operating level at the ADC increases as processing bandwidth increases. The ADC cannot be operated to full scale, in practice.
Evaluating System Results
It is proposed that the Walden and Schreier ADC FOMs can be used to establish system FOMs to compare performance vs. power trades for RF-to-ADC cascades. The goal is to sweep parameters and spot best value performance normalized for DC power at the system level.
Here, the FOMs are presented while:
- Varying RF: digital summation, from all-digital to all-RF
- Varying ADC ENOB, linearity, and DC power
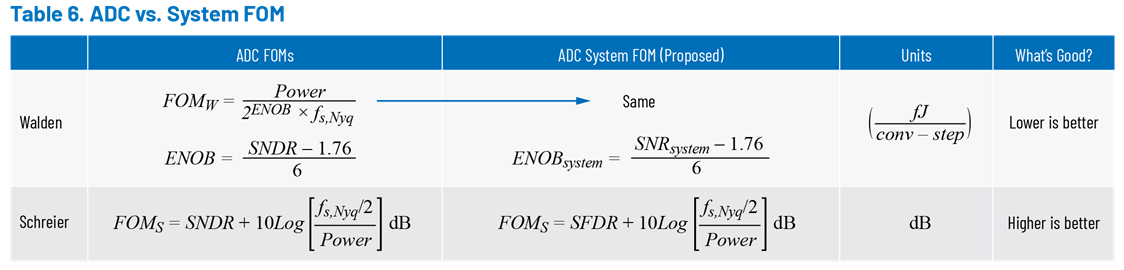
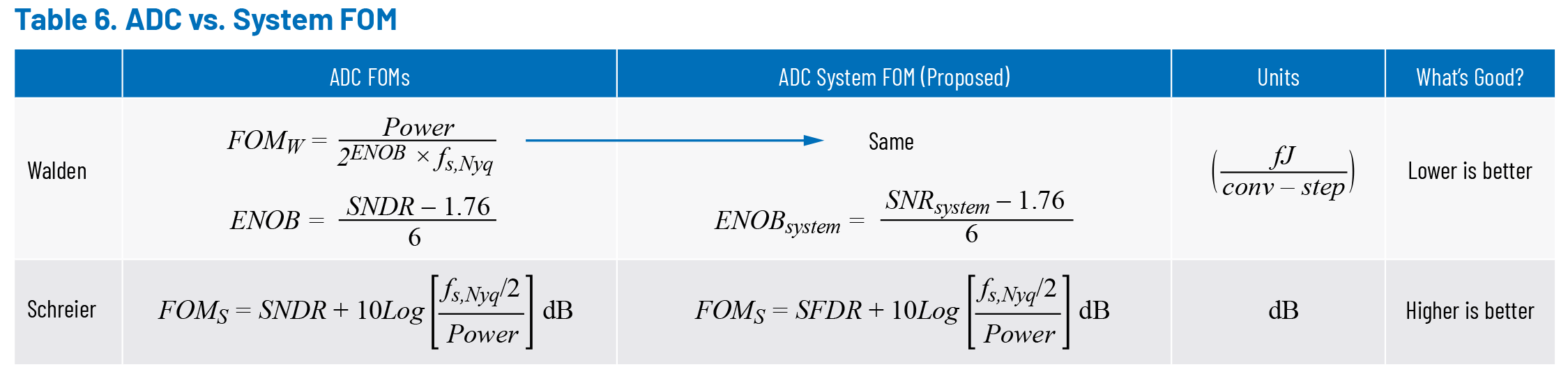
We suggest the Schreier FOM can be modified by swapping in SFDR for SNDR to make a FOM that reflects two-tone linearity performance. Figure 11 and Figure 12 plot the system FOMs vs. RF: digital sum ratio.

T = 290 K and full scalesystem.input is the input-referenced full scale.


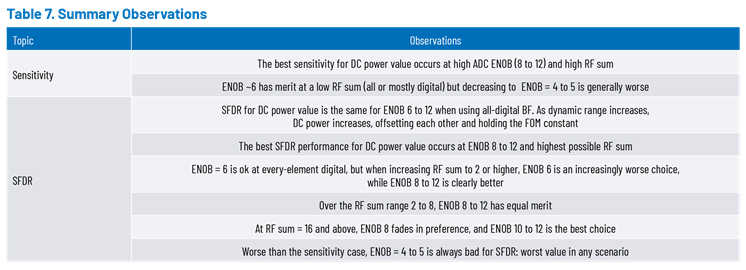
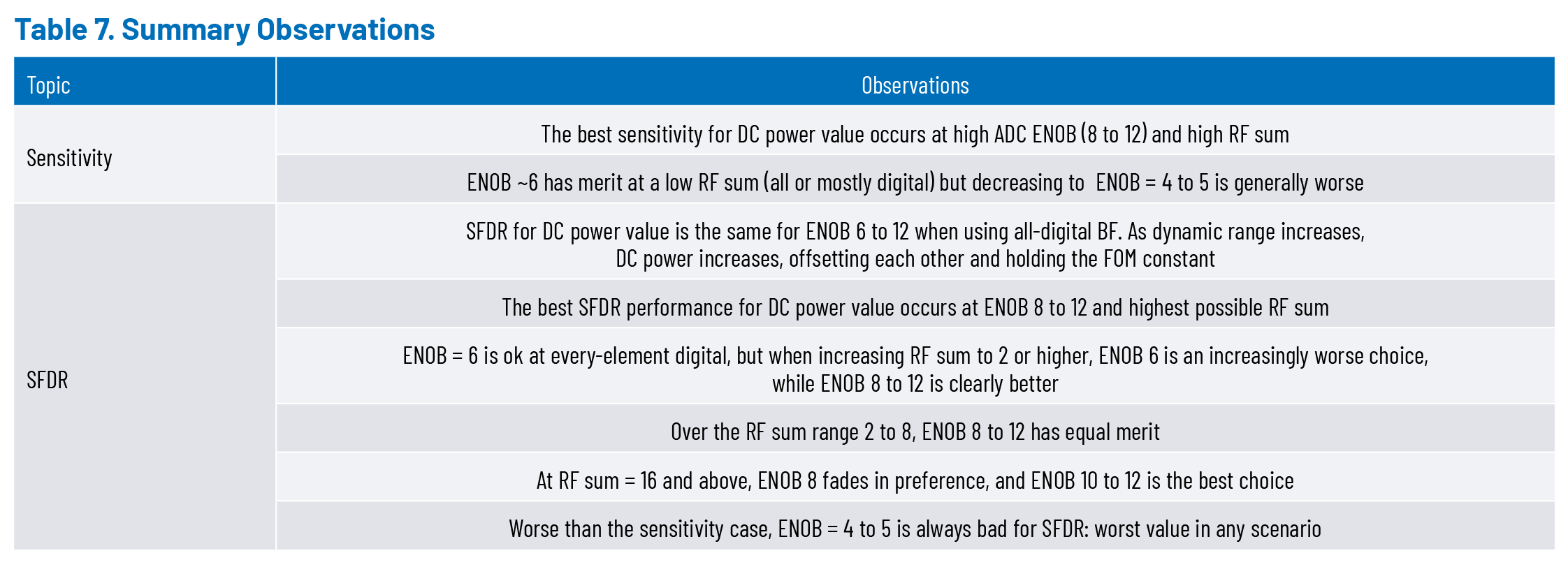


The phrase “best value” is meant in the sense of best return (that is, performance) for a given cost (that is, DC power burn). The FOMs assist in drawing best value conclusions because they normalize performance against DC power burn (see Table 7). Sensitivity observations are drawn from Walden (Figure 11, lower is better) and SFDR comments are drawn from Schreier (Figure 12, higher is better).
Conclusions
Performance-critical applications like radar phased arrays need to deploy the optimal balance of sample rate, dynamic range, and DC power. Overprioritizing any one of these will result in a suboptimal (or simply bad) solution.
The era of 20 GSPS to 100+ GSPS ADC sampling is here, but higher sampling is achieved at a cost to the other two critical performance attributes in the FOM triad—DC power and dynamic range (ENOB). High sample rates are not a design miracle, but a chosen prioritization of sample rate at the expense of higher DC power and lower ENOB. In many cases the optimal ADC for a phased array system will prioritize dynamic range and DC power, with just a high enough sample rate for frequency planning efficiency and oversampling gain.
High dynamic range, high sample rate data converters with ENOB ~8 are popular choices for phased array radar because they offer the best compromise between dynamic range and DC power. Be careful how SNDR (ENOB) is defined and make sure to consider two-tone intermodulation performance with SNDR. Phased array radar ADCs also need high linearity (that is, IP3 > 22 dBm). When evaluating SNDR, know whether it includes interleave spurs, and make sure spectral regions aren’t cherry-picked.
You need to have a mission-critical reason to do high dynamic range, every-element digital beamforming. It comes at a steep DC power penalty. The arrays with the best balance of performance and DC power use a hybrid scheme, which is a combination of RF beamformed subarrays feeding distributed DAC/ADC nodes that are digitally beamformed. If beam attributes allow it, a small subarray of RF beamforming in front of each ADC is helpful in improving performance and lowering DC power. RF beamforming is highly recommended for improving SFDR and sensitivity at lower DC power and providing blocker mitigation using beam null steering, to name a couple of benefits. Extra power is burned to achieve the software-defined adaptability of fully digital beamforming.
Over the next 5 to 10 years, every-element digital phased array will ramp in technology readiness and viability at increasingly better performance. To get there, new state-of-the-art ADCs will put a higher emphasis on lowering DC power while maintaining sample rate and ENOB. ADC sample rate capability will continue to push higher and grab headlines but might benefit wideband applications like EW more than phased array. The phased array market will figure out a sample rate sweet spot (10 GSPS to 20 GSPS?) and then the market winners will provide the best ENOB at lowest power.
著者について
Benjamin Anninoは、アナログ・デバイセズのアプリケーション・ディレクタです。航空/防衛ビジネス・ユニットを担当しています。2011年にHittite Microwave(現在はアナログ・デバイセズに統合)に入社。2014年にアナログ・デバイセズに転籍しました。それ以前は、Raytheonで様々なレーダー技術に従事。ダートマス大学で電気工学の学士号、マサチューセッツ大学ローエル校で電気工学の修士号、マサチューセッツ大学アマースト...
{{modalTitle}}
{{modalDescription}}
{{dropdownTitle}}
- {{defaultSelectedText}} {{#each projectNames}}
- {{name}} {{/each}} {{#if newProjectText}}
-
{{newProjectText}}
{{/if}}
{{newProjectTitle}}
{{projectNameErrorText}}